MU123 Unit 3 Notes

1 Natural numbers

Positive integers 1, 2, 3, 4... Some include zero, some not.

1.1 Multiples

A result of multiplying a natural number by natural number. E.g. multiples of 8 are $8, 16, 24, 32$

Thus multiples are numbers into which 8 divides exactly.

Common multiple

is a multiple of all the numbers in question. Lowest common multiple (LCM) is the smallest number that is a multiple of numbers in question

1.2 Factors

A natural number that divides a number exactly into a second natural number, e.g. 2 is factor of 10. Alternatively we can say 10 is divisible by 2.

Related to multiples since 2 is a factor of 10 is same as 10 is a multiple of 2.

All natural numbers > 1 have at least two factors - itself and 1.

Factors can be arranged into factor pairs - $1,10$; $2,5$.

Strategy to find factors of a number

• Try 1,2,3,4... in turn. When factor is found, write it down with the other factor in the pair
• Stop when you reach already found factor pair

Common factors

is a factor of all the numbers in question. The highest common factor (HCF) is the largest number that is a factor of numbers in question.

1.3 Prime numbers

Natural numbers that have exactly two factors - 1 and itself, e.g. $2, 3, 5, 7, 11, 13, \ldots$.

Sieve of Eratosthenes

An algorithm for finding all the prime numbers up to a certain number.

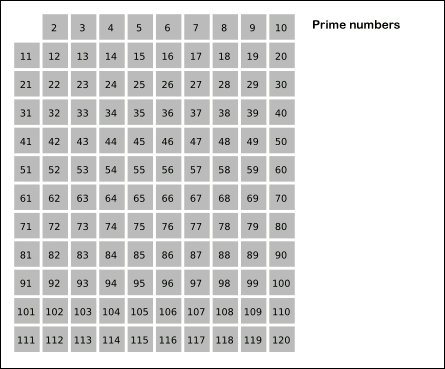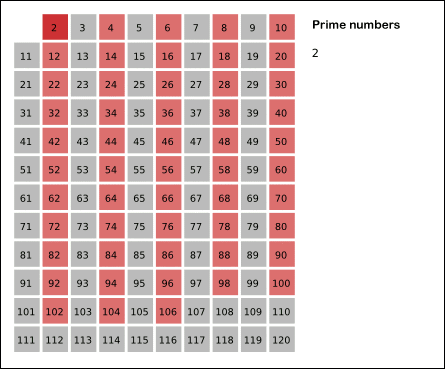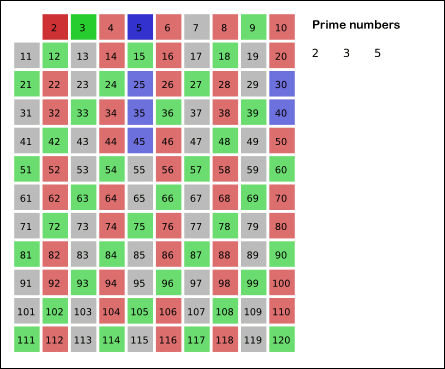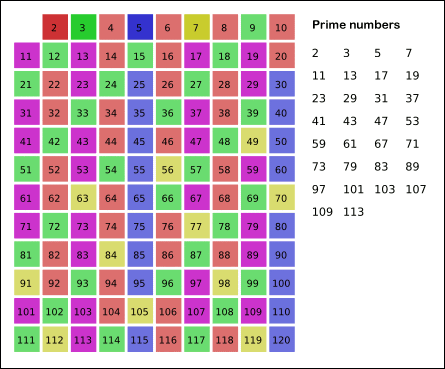
• Create a range of $2..n$ where $n$ is a certain number
• Mark all the numbers that are multiples of 2 and are $>= 2^2$
• Move to next unmarked number - 3 and mark its multiples that are $>= 3^2$
• ...
• All the unmarked numbers are primes

Python impelementation src:

def SieveOfEratosthenes(n):
 
    # Create a boolean array 
    # "prime[0..n]" and initialize
    #  all entries it as true.
    # A value in prime[i] will
    # finally be false if i is 
    # Not a prime, else true.
    prime = [True for i in range(n+1)]
    p = 2
    while (p * p <= n):
 
        # If prime[p] is not 
        # changed, then it is a prime
        if (prime[p] == True):
 
            # Update all multiples of p
            for i in range(p * p, n+1, p):
                prime[i] = False
        p += 1
 
    # Print all prime numbers
    for p in range(2, n+1):
        if prime[p]:
            print p,

Resources

• https://www.geeksforgeeks.org/sieve-of-eratosthenes/
• https://cp-algorithms.com/algebra/sieve-of-eratosthenes.html
• https://www.baeldung.com/cs/sieve-of-eratosthenes

Mersenne primes

$$ 2^n - 1 ~\text{for natural number} ~n $$ It can be proven that if $2^n - 1$ is prime then $n$ must be a prime.

Resources

• https://en.wikipedia.org/wiki/Mersenne_prime
• https://www.mersenne.org/
• https://primes.utm.edu/mersenne/

1.4 Prime factors

A natural number > 1 that is not prime is called a composite number, e.g.: $4, 6, 8, 9, 10\ldots$. A composite number can often be written as a product of even more factors forming a factor tree:

Hence we can say $360 = 2^3 \times 3^2 \times 5$. All of the factors here are primes.

I could've started with different nodes, e.g. $360 = 10 \times 36$, but the result would've been the same.

The process of writing a natural number as a product of factors > 1 is called factorisation.

The fundamental theorem of arithmetic

Every natural number > 1 can be written as product of prime numbers in just one way.

The prime factorisation of a natural number is the product of prime factors that is equal to it, e.g.: $$ \begin{aligned} 2 &= 2 \qquad 3 = 3 \qquad 4 = 2^2 \\ 5 &= 5 \qquad 6 = 2 \times 3 \qquad 7 = 7 \\ 8 &= 2^3 \qquad 9 = 3^2 \qquad 10 = 2 \times 5 \end{aligned} $$

Because of the fundamental theorem of aithmetic we can say that prime numbers are the building blocks of natural numbers.

For prime factorisation it may be helpful to be systematic and follow: $$ \text{the smallest possible prime} \times \text{a number} $$ And iterate for each composite number in the factor tree, e.g.:

There's no other way to find prime factors of a number $n$ then trying all the primes < $n$. So multiplying two large primes is a quick process but expensive to reverse. This fact is a base to secure encryption systems.

Finding LCM and HCF using prime factors

• Find the prime factorisation of the numbers.
• To find the LCM, multiply together the highest ower of each prime factor occuring in any of the numbers.
• To find the HCF, multiply together the lowest power of each prime factor common to all the numbers.

1.5 Powers

Index laws

So far I've used these $$ \begin{aligned} a^m \times a^n &= a^{m+n} \qquad \frac{a^m}{a^n} = a^{m-n} \\ (a^m)^n &= a^{mn} \\ (a \times b)^n &= a^n \times b^n \qquad (\frac{a}{b})^n = \frac{a^n}{b^n} \end{aligned} $$

2 Rational numbers

Include natural numbers among others.

Is a number that can be written as a fraction of two integers: $\frac{integer}{integer}$.

Following numbers can be written in this form:

• Fraction as $\frac{3}{4}$
• Mixed number as $5\frac{3}{4}$ since it can be converted to top-heavy fraction: $\frac{23}{4}$
• Whole number: $7 = \frac{7}{1}$
• Decimal number with finite number of digits after decimal points: $0.42 = \frac{42}{100}$
• Some decimal numbers with infinite numbers after decimal point: $0.333\ldots = \frac{1}{3}$
• The negative of any above: $-4 = \frac{-4}{1}$

Irrational numbers

• $\pi$
• $\sqrt{2}$

Rational numbers as decimals

Every rational number can be converted to decimal, e.g.: $\frac{5}{8} = 5 \div 8 = 0.625$ There are two possible outcomes of the division:

• decimal number with finite digits after d.p. - terminating decimal
  • $0.625$ is terminating
• decimal with block of indefinitely repeating digits after d.p - recurring decimal
  • $\frac{2}{3} = 0.6666\ldots$ is recurring
  • Can be denoted with $0.1\overline{23}$.

Thus rational numbers can be redefined as

Decimal numbers that are terminating or recurring.

I can convert terminating to fraction so I can prove first part of the statement but I can't prove the second yet.

2.2 Adding and subtracting fractions

• Only when there's a same denominator - fractions are of a same type.
• When there's different denominator - find common one (LCM).
• Always simplify.

2.3 Multiplying and dividing fractions

Multiplying

• Multiply the numerators together and multiply the denominators together.

Dividing

• Multiple the fraction by its reciprocal

Reciprocal

A number and its reciprocal multiply together to give 1. E.g.: $0.25 ~\text{is rec of} ~4$,since $0.25 \times 4 = 1$ $\frac{3}{2} ~\text{is rec of} ~\frac{2}{3}$,since $\frac{3}{2} \times \frac{2}{3} = 1$

Alternatively, it is 1 divided by given number.

To find a reciprocal of fraction turn it upside down. E.g.: $\text{rec of}~\frac{3}{4}~\text{is}~\frac{4}{3}$

2.4 Negative indices

The previous positive power of a number $n$ is equal to current positive power divided by $n$, e.g.: $4^3 = \frac{4^4}{4}$

Thus $4^0$ has to be a number I get when dividing $\frac{4^1}{4} = 1$. Similarly $4^{-1}$ has to be a number I get when dividing $\frac{4^0}{4} = \frac{1}{4}$.

We can build whole scale of the pattern

The meaning of negative and zero indices works with all the index laws I've seen so far.

Index laws can be expand by following rules based on previous observations:

• A non-zero number raised to the power zero is 1: $$ a^0 = 1 $$
• A non-zero number raised to a negative power is the reciprocal of the number raised to the corresponding positive power: $$ a^{n - 1} = \frac{1}{a^n} $$

Interestingly: $$ a^{-1} = \frac{1}{a} $$ Hence, raising a number to the power of $-1$ is the same as finding its reciprocal.

E.g.: $$ (\frac{2}{3})^{-1} = 1 \times \frac{3}{2} = \frac{3}{2} $$

2.5 Scientific notation

• Also called standard form.
• A number between 1 and 10 (excluded) multiplied by a power of 10.

3 Irrational numbers and real numbers

3.1 What is an irrational number?

Decimals with infinite number of digits afterthe decimal point but no repeating block of digits.

Following number is not terminating nor recurring: $$ 0.010,010,001,000,010,000,01\ldots $$

TODO: What if the repeating block is just really big?

Here $x$ is not rational:

$$ \begin{aligned} x^2 = 2 \\ \sqrt{2} = x \end{aligned} $$

The fact that $\sqrt{2}$ is irrational can be proven by contradiction followingly.

Suppose there's a rational number whose square is $2$. Hence it can be written as $$ \frac{\text{integer}}{\text{integer}} $$ Consider it being cancelled down to the simplest form as: $$ \frac{m}{n} $$ So $m$ and $n$ don't have any common factor. The square of $\frac{m}{n}$ is 2, so: $$ \begin{aligned} (\frac{m}{n})^2 = 2 \\ \frac{m^2}{n^2} = 2 \end{aligned} $$ It follow that $$ \begin{aligned} \frac{m^2}{n^2} \times n^2 = 2 \times n^2 \\ m^2 = 2n^2 \end{aligned} $$ $2n^2$ has to be even since it's a product of 2 and whole number. Meaning $m^2$ has to be even too, that means $m$ has to be even as well. That means that $m = 2r$ for some integer $r$. When substituting to the original equation we get: $$ \begin{aligned} (2r)^2 = 2n^2 \\ 4r^2 = 2n^2 \\ \end{aligned} $$ It follow that $$ \begin{aligned} \frac{4r^2}{2} = \frac{2n^2}{2} \\ 2r^2 = n^2 \end{aligned} $$

The integer $n^2$ is even hence $n$ has to be even too. So both $m$ and $n$ are even. But that's impossible since $m$ and $n$ don't have any common factor.

The irrational numbers together with the rational numbers form the real numbers.

I can think about various categories of numbers as layers:

Real(
	Irrational,
	Rational(
		Integers(
			Natural
		)
	)
)

plus complex, that will come later on.

3.2 Roots of numbers

A number that when multiplied by itself gives the original number. Every positive number has two square roots - a positive one and a negative one.

Square roots of two numbers can be used to find the square root of the product or a quotient.

For example, numbers 16 and 49 have square roots of 4 and 7. I can apply index law $(a \times b)^n = a^n \times b^n$ to those: $$ \begin{aligned} (4 \times 7)^2 = 4^2 \times 7^2 \\ (4 \times 7)^2 = 16 \times 49 \end{aligned} $$ That implies: $$ \sqrt{16 \times 49} = \sqrt{16} \times \sqrt{49} $$

This can be generalized into following laws: $$ \sqrt{a \times b} = \sqrt{a} \times \sqrt{b} \qquad \sqrt{\frac{a}{b}} = \frac{\sqrt{a}}{\sqrt{b}} $$

Obviously, these laws apply to any root.

3.3 Surds

Numbers that are not perfect square have irrational roots. Hence following roots are irrational: $$ \sqrt{7}, \sqrt{8}, \sqrt{10}, \sqrt{11} $$ Since such number can be only approximated as a decimal it should be left as is in calculations, e.g.: $$ x = 2 \times \sqrt{5} $$ such expressions are called surds - it contains one or more irrational roots of numbers. Surds are usually written concisely as formulas, number before surd when multiplied: $$ y = 2\sqrt{5} $$ In the simplest form: $$ \sqrt{12} = \sqrt{4 \times 3} = \sqrt{4} \times \sqrt{3} = 2\sqrt{3} $$ This is obviously possible when some of a numbers under the root are perfect squares. Thus the goal when simplifying is to find some perfect square multiplied some other number giving the original number bellow the root.

Multiplying roots

• Multiply numbers together
• Multiply roots together
• Simplify the root

Dividing roots

Use following laws $$ \sqrt{a \times b} = \sqrt{a} \times \sqrt{b} \qquad \sqrt{\frac{a}{b}} = \frac{\sqrt{a}}{\sqrt{b}} $$

Sometimes, I should expand an integer to a multiplication of surds in order to simplify the quotient: $$ \frac{2}{\sqrt{2}} = \frac{\sqrt{2} \times \sqrt{2}}{\sqrt{2}} = \frac{1 \times \sqrt{2}}{1} = \sqrt{2} $$

Adding and substracting roots

It's usually not possible to add or substract different roots, thus $$ \sqrt{a} + \sqrt{b} \not= \sqrt{a + b} $$

I can only add or substract roots that are the same.

To simplify surds

• Simplify roots of integers with square factors.
• Simplify products and quotients of roots.
• Add or subtract roots that are the same.

3.4 Fractional indices

Index laws can be applied to fractional indices too, e.g. let's consider the power $5^\frac{1}{2}$

By applying $$ a^m \times a^n = a ^{m + n} $$ I get $$ 5^\frac{1}{2} \times 5^\frac{1}{2} = 5^{\frac{1}{2} + \frac{1}{2}} = 5^1 = 5 $$ Hence $$ 5^\frac{1}{2} = \sqrt{5} $$

It can be generalized in the following index law $$ a^\frac{1}{n} = \sqrt[n]{a} $$

This rule toegher with other index laws can be used to give a meaning to any fractional index.

E.g. in combination with $$ (a^m)^n = a^{mn} $$

I can do following operation $$ 5^\frac{4}{3} = 5^{\frac{1}{3} \times 4} = (5^{\frac{1}{3}})^4 = (\sqrt[3]{5})^4 $$

This combination can be generalized to yet another index law $$ a^\frac{m}{n} = (\sqrt[n]{a})^m $$

Interestingly the square root laws I saw before: $$ \sqrt{a \times b} = \sqrt{a} \times \sqrt{b} \qquad \sqrt{\frac{a}{b}} = \frac{\sqrt{a}}{\sqrt{b}} $$ Can be deduced by applying $n = \frac{1}{2}$ to following index laws: $$ (a \times b)^n = a^n \times b^n \qquad (\frac{a}{b})^n = \frac{a^n}{b^n} $$

Irrational indices are also valid but I will encounter those later on.

4 Ratios

4.1 What is a ratio?

Comparition of multiple ordered quantities, e.g.: $3:5 ~ 2:10:7$

Sometimes it's useful to convert a ratio to a $number:1$ form to e.g.

• Compare multiple ratios.
• Find approximate ratios. To reach such a form I can divide both numbers by second number, e.g.: $$ 7 : 11 = \frac{7}{11} : \frac{11}{11} = 0.636 : 1 ~\text{(3 d.p.)} $$